What is the Philosophy of Physics?
From Physics to Philosophy and Back Again
The philosophy of physics is a teeming collection ideas that forms one of the largest branches of the philosophy of science. There is no sharp boundary between the aspects of the field that belong to philosophy, and those that belong to physics. However, there has been a historic interplay between these two kinds of fields.
Historically, there was no division between the study of philosophy and physics. In terms of how the subjects are now studied in universities, Descartes, Newton, and Leibniz all contributed as much to philosophy as they did to physics.



Indeed, the term "metaphysics" or "after physics" was not introduced to distinguish it from physics in any way; Metaphysics was placed after Physics on the bookshelf by the early publisher of Aristotle's works Andronicus of Rhodes, who coined the name.

In the 20th century, the philosophy of physics is remarkable for having grown up hand in hand with modern physics. The development of 20th century physics deeply influenced analytic philosophy.
| Physical Theory | Philosophy It Influenced |
|---|---|
| Statistical Mechanics | Philosophy of Probability, Realism, Mereology |
| Thermodynamics | Arrow of time, Reduction and Emergence |
| Quantum Mechanics | Logic, Indeterminism |
| Relativity and Gravitation | Space, Time, Geometry, Positivism |
Among the most dramatic changes was the complete overturning Kant's philosophy of space. Kant thought that the application of Euclid's axioms to space was a known fact, through what Kant called "synthetic a priori" reasoning.
Plausible though it may seem, Nature did not agree: spacetime was discovered to be curved and non-Euclidean in the 20th century, in accordance with general relativity, but not Kant. And although some philosophers have proposed revisions of Kant's doctrine that are relativity-compatible, Kant's views never regained their previously dominant status in the philosophy of space and time.

Conversely, modern philosophy of physics has played an inextricable role in the development of modern physics. Einstein was deeply influenced by empiricism and by the philosophical ideas of Mach in the development of relativity theory. The developers of quantum mechanics were centrally concerned with understanding the nature of objectivity and the role of observation in determining the nature of reality.
But perhaps even more importantly, physics after the 1960's became centrally concerned with its philosophical foundations. Many problems arose in the specific physical sciences that philosophers and physicists have worked together to solve.
Here are a few representative examples of the kinds of problems philosophers of physics engage with.
Specific Disciplines
1. Space and time. How should we understand the geometry of space? The nature of motion through space? How many dimensions of space are there and why? In what sense is time directed, and how does its passage occur? What new interpretive lessons about space, time and gravity must be drawn in the face of relativity theory?
2. Quantum Theory. What is the role of a measuring observer in the development of matter? What is entanglement, how pervasive is it, and how does it constrain experimentation? How does quantum theory change how we think about the transmission of information? Is matter fundamentally made of particles or fields?
3. Thermal Physics. How and why do things happen asymmetrically in time? How are large, visible phenomena related to their microscopic constituents, and are they described by fundamental laws? How are the laws of thermal phenomena related to other theories? How do we understand and interpret phenomena in which the microscopic laws of physics seem to break down, such as during phase transitions and spontaneous symmetry breaking?
4. Quantum Gravity. In what sense should it be possible to think of gravity as a branch of quantum theory, and why? What new constraints does quantum theory impose on the nature of space and time? What aspects of current theories should we expect to carry over to a theory of quantum gravity, and what aspects shouldn't? What are the empirical adequacy conditions for a quantum theory of gravity?

5. General Philosophy of Physics. When and how is a theory of physics confirmed? How does physics produce knowledge from experimentation? What is a law of physics? What sort of realism is appropriate for modern physics? How do the different physical theories relate to each other? What is an interpretation of physics, and when is one needed?
This last question is the question of an interpretation of physics. It intersects many different areas of the philosophy of physics, and so it is a nice example for us to take up of philosophy of physics in action.
Interpreting a theory of physics
No matter how fluent of a language-speaker you are, a language is a hard thing to understand. The literal meaning of a word or phrase is not always obvious.
- A 'fat chance' is the same as a 'slim chance'.
- You park your car in the driveway, and drive your car in the parkway.
Other phrases offer multiple possible interpretations, such as the proverb, "A rolling stone gathers no moss".

Our best theories of physics in some ways different than common language. For example, they make much more frequent use of mathematical concepts. But there are still many situations in which we don't know (or don't agree) on what some aspect of a physical theory means. In such cases, an interpretation is needed.
The interpretation of Classical Mechanics
Interpretive questions already appear in the early theories of Newton and his followers known as Classical Mechanics. Many interpretive questions were asked during the development of this theory. But perhaps the most basic was: What kinds of things does Classical Mechanics describe? Of course we know from experience that it describes the mechanical motion of things like orbiting bodies, projectiles and bouncing springs.

But what properties do these bodies share by the lights of Classical Mechanics? What does Classical Mechanics say the world is like?This is a question about the interpretation of Classical Mechanics.
One might start by looking at a canonical textbook account of Newton's laws, and the most famous representative among them:
Force = Mass x Acceleration.
This law appears prominently as the 2nd Law in Newton's Principia.

The fact that only forces and masses in motion appear in Newton's laws suggests an interpretation in which the "furniture" of the universe consists of forces and masses in motion.
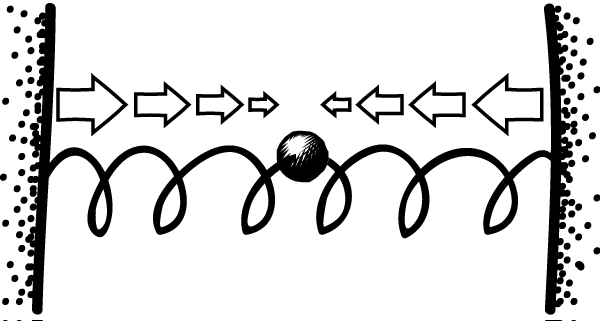
For example, the little bob in the image above is a mass, and the spring provides a force. Of course, this description leaves out all the parts that make up the spring and the two walls. But on this interpretation, everything can be ultimately described by masses and forces of some kind or another.
This simple interpretation leads to a number of difficulties that deeply troubled those who thought about the philosophical foundations of Classical Mechanics during its heyday.
First, a number of things described by Classical Mechanics neither forces nor masses:
- Waves and fields, such as water waves, sound waves, and vector or temperature fields.

- Continuum surfaces making up a solid object or a liquid.
- Beams of light that lack any mass, but are not forces either.


There were many theories developed, such as wave mechanics, continuum mechanics, and eventually electromagnetism, according to which these kinds of phenomena did not require particulate masses, but could themselves be fundamental. Indeed, it did not seem possible to express many of the central properties of such phenomena in terms of just particulate masses and forces. There were even approaches to Newton's mechanics, developed by Lagrange and by Hamilton, which redescribed the same phenomena without any mention of forces or masses.
Stilll, some suggested that all such phenomena might be reducible to the motion of particulate masses. Robert Boyle gave expression to this view in 1772:

"whereas those other philosophers give only a general and superficial account of the phaenomena of nature... both the Cartesians and the Atomists explicate the same phaenomena by little bodies variously figured and moved" (Boyle 1772)
But the atomist interpretation of Classical Mechanics was a matter of great debate, only reached a resolution in the 20th century when the atomic hypothesis was finally (in a way) accepted.
Notice that which interpretation we choose can have consequences for how we evaluate the success of a theory. For example, suppose 19th century scientists were to discover that the atomic hypothesis is correct. Would be evidence in favour of Classical Mechanics, or evidence against it? According to the atomist interpretation it is evidence in favour; otherwise it may not be.
A consequence of this is that our interpretation also determines whether or not we have reason to be a realist about a theory. We will return to this point shortly.
Pristine interpretations
In her recent Lakatos award-winning book on the nature of quantum theory, Laura Ruetsche provides a much more careful description of what an interpretation is. Moreover, she points out that there are really two kinds of interpretations of a physical theory that one might be interested in.
Laura Ruetsche's lecture at the LSE Lakatos Award conference
The first type of interpretation, which she referred to as "pristine", is what many philosophers of physics mean when they refer to an interpretation of a theory.
Pristine Interpretation
We implicitly gave a pristine interpretation of Classical Mechanics above when we suggested that it consists of forces and masses. This interpretation was given using only terms appearing in the laws. It made no reference to any particular "contingent" facts, such as the fact that the Earth has one moon.
A pristine interpretation is a very clean and simple way to think about what is required of an interpretation of physics. However, to assume that every interpretation is pristine is a little bit blunt. Ruetsche likens this interpretation to John Belushi's role as the Samurai TV Repair Man, who applies the same procedure to every broken TV: smash it with his sword.
Although a pristine interpretation may be sufficient in some cases, Ruetsche argues that it might not be sufficient in all cases. Sometimes, an interpretation that draw on particular facts might be needed. Ruetsche refers to this second kind of interpretation as "adulterated".
Adulterated Interpretation
The central distinction that Ruetsche has drawn is that adulterated interpretations are conditioned on contingent facts, while pristine interpretations are not. Thus, a pristine interpretation should not depend on facts like "there are no gold masses larger than 8 tons", but does depend on facts like "there are no uranium masses larger than 8 tons". An adulterated interpretation can depend on either.
The aim of an interpretation in either case, according to Ruetsche, is simply to allow a theory of physics to explain the way things are. She does not subscribe to any particular account of explanation, but suggests it involves a number of things like prediction, unification, or whatever you else you like.
Since both pristine and adulterated interpretations can achieve these things, Ruetsche argues that both should be available to the philosopher of physics.
Moreover, there are situations in which an adulterated interpretation is to be preferred. We turn to such situations next.
Ruetsche's Problem of Multiple Interpretations
Ruetsche points out that the interpretation of physics can pose a special problem for scientific realism. This problem can be summarised as the following argument.
The Problem of Multiple Interpretations
- One should be a realist about a theory of physics only if there is a consistent set of reasons to believe that theory.
- If there is a consistent set of reasons to believe a theory, then these reasons should not depend on one's interpretation.
- If we should be concerned with practical applications, then the reasons to believe a theory do depend on one's interpretation of that theory.
- We should be concerned with practical applications.
- Therefore we should not be a realist about such a theory of physics.
The problem with the conclusion is that this antirealism defeats the entire aim of an interpretation, in denying that there is anything meaningful to be said about what the central terms of a theory mean.
The argument above is valid. So, we can only deny the conclusion by denying at least one premise. Let's consider each of them.
- The first premise expresses that philosophy of physics should be rational, in the sense that we should only be realists when we have a reason to believe the theory is correct. This seems very plausible.
- The second premise expresses that an interpretation is "extra" in that it is not a part of why we believe a theory. For example, in Classical Mechanics, it would say that our reasons to believe Force = Mass x Acceleration are independent of interpretive claims like atomism. In general, the reasons to believe a theory should not depend on an interpretation (according to this premise).
- The third premise is a specific claim about the physical sciences. Ruetsche claims that it may not always be true, but that it is at least true in the case of quantum field theory. It may also be true in the case of Classical Mechanics as well: as we saw above, one's atomism may give one more or less reason to believe in Classical Mechanics.
- The fourth premise is a matter of how one approaches the philosophy of physics.
According to Ruetsche, advocates of the Pristine Interpretation can deny premise 4 in order to escape the problem. A truly "pristine" interpretation should not be considered with the many contingent factors entering into the practical application of physics.
However, many may find this response unsatisfying, if the philosophy of physics owes some attention to the practice of physics. In that case, Ruetsche recommends that we accept the adulterated interpretation. And there is a way to escape the problem on that view, too: we can deny the second premise.
Thus, Ruetsche suggests, we should view our reasons to believe a theory of physics as tied up with a plurality of interpretations, some of which give us more reason and others less reason to believe that theory.
What you should know
- Some of the major categories of philosophy of physics
- What an interpretation of physics is
- The difference between Pristine and Adulterated interpretations
- Ruetsche's problem of multiple interpretations