Introduction to Markets
Markets are tantalising places, and have been since ancient times. They often radiate a chaotic, eccentric atmosphere, while also allowing the transfer and creation of large amounts of wealth.

"Market at Jaffa" by Gustav Bauernfeind
Let's begin with a crash course in financial markets: first from a physics perspective, and then in terms of the concrete structures that make them up. We'll then turn to how it is, from a physics perspective, that a theory about such structures might successfully represent the world.
Market dynamics: A first physical perspective
In much of physics, including quantum mechanics, one takes a complex physical scenario and reduces it down to a description of the possible states, together with an account of how those states change over time. For example, a pair of planets can be located in various possible states, and their changes can be described by Newton's law of gravity.
Facts about two planets reduced to a set of possible states and an account of how they change.
One can take a similar perspective on markets. At its core, a market is in fact a remarkably simple structure: a market is a collection of quantities that can be traded for a given price. In early societies, useful minerals like obsidian or copper could be traded in a market for food or weapons. In colonial American markets you could trade beaver pelts for rifles and horses. At Tesco you trade the national currency for groceries. For each item in a market, we can assign a pair of real numbers:
\[(q,p)\]where \(q\) is the quantity of that item, and \(p\) is a price associated with that quantity. For a market in which there is a number of items \(n\) available, a possible market event is described by an ordered set of quantities and prices,
\[(q_1,q_2,\dots,q_n; p_1,p_2,\dots,p_n).\]Such an event describes what is happening in the market at any given time. For example, perhaps only apples and pears are available to be sold in some market. Then a possible market event is the sale of 2 apples for £4 and 1 pear for £3 pounds, given by \((q_1,q_2;p_2,p_2) = (2,1;4,3)\). The space of all possible trade events consists in all the possible \(2n\) values of that can be assigned to this ordered set. We might call such a set "trade space".

The word dynamics refers to motion. So, the phrase market dynamics refers to the way that a market "moves" through trade space. In particular, it is a description of trade events that occur over the course of time. At any given moment, some set of of purchases and sales is happening in the market, corresponding to some fixed quantities and prices. At a later moment another set of purchases and sales occurs, and then another, and then another. The succession of all these trade events is the market dynamics.
If only one could find a way to reliably predict the market dynamics, one could make a great deal of money. There are ways of doing this. Unfortunately, as we shall see in this and future lectures, it is not such a simple task.

A fortune-teller attempts to predict the future of a market.
In the coming lectures, we will consider how some of the ideas we've learned from physics might be used to help us make predictions about market dynamics in trade space. Since the particular trade space we are interested in does not involve apples and pears, but special kinds of products appearing in financial markets, let's begin with the two basic types of financial products: (1) elementary, and (2) derivative.
Elementary Financial Products
Finance is the study of markets that trade in financial products. Unlike in ordinary markets, in financial markets you don't have to own something to sell it. This is sometimes called short selling. We will see examples of it in play soon. Tou might not interact with them much just yet, financial markets are raging all around you. You can enter the market by opening a trading/brokerage account via your bank or an online brokerage firm, which allows you to view, buy and sell financial products.
There is a vast array of different kinds of financial products available in a financial market. However, they are all built out of simple components, called elementary financial products, where we use "elementary" in the sense of "basic/fundamental", rather than "easy". Elementary financial products are identifiable with an item whose quantity and price at a given moment can be directly determined, independently of the quantity and price associated with any other product. For example:
| Stock - a fraction ownership of a company. |
| Bond - a fraction of a loan, like an IOU, usually owed by a company or government. |
| Cash - the good old dosh, dough, or cheddar, in some currency. |
| Wheat - one of the oldest financial products, available for trade today in commodities exchange markets. |
| Silver - another ancient commodity, traded alongside gold and other metals in today's commodities exchanges. |
These products are "elementary" in the sense that their quantity and price is not tied by definition to another product.
Note that this is does not mean that elementary financial products don't influence each other: they do. For example, a rise in the price of the oil (a commodity) can have a huge impact on the price of an airline company's stock in the future. But that is a matter of market dynamics. To know the price of an airline stock in contrast is a fact about trade space. And it is not the case that you must know the price of oil in order to know the current price of the airline's stock at a given moment. This is characteristic of elementary financial products. To take a trivial example: a £10 note has a value of — you guessed it — 10 pounds, independently of any other prices.

Elementary Securities
The elementary financial products of a stock market are stocks and bonds, sometimes referred to as elementary securities. Much of our discussion here will be about securities, so it is worth being clear on exactly what they are.
A bond is a portion of an IOU, to be paid some day by someone who received a loan. Bonds are not issued by individuals, but rather by institutions like governments and companies, for the purposes of some sort of investment.
A bond splits a loan up into portions that may be bought and sold, allowing the purchaser to make money on the interest.
And, a stock is a fraction of ownership of a company. For example, as of 2017, Apple Inc. has been split into about 5.2 billion shares. The largest shareholders are Arthur Levinson (chairman of the board for Apple) and CEO Tim Cook, each of whom own about a million shares. You can purchase a share from the NASDAQ stock market during market hours using a brokerage account, for something around the going market price, which you can find (expressed in US Dollars) by searching for their stock code AAPL.

Derivative Financial Products
The other kind of financial product that you will find in the market is a derivative. As the name implies, its price is derived from more elementary securities. There are many, many kinds of derivatives sold in financial markets, with names like futures, forwards, options, swaps, and the infamous mortgage-backed securities associated with the collapse of the world economy in 2008. We will only learn about a few of them in this course. Let's start with the simple example of a "future contract".

Someone interested in futures contracts: An airline company
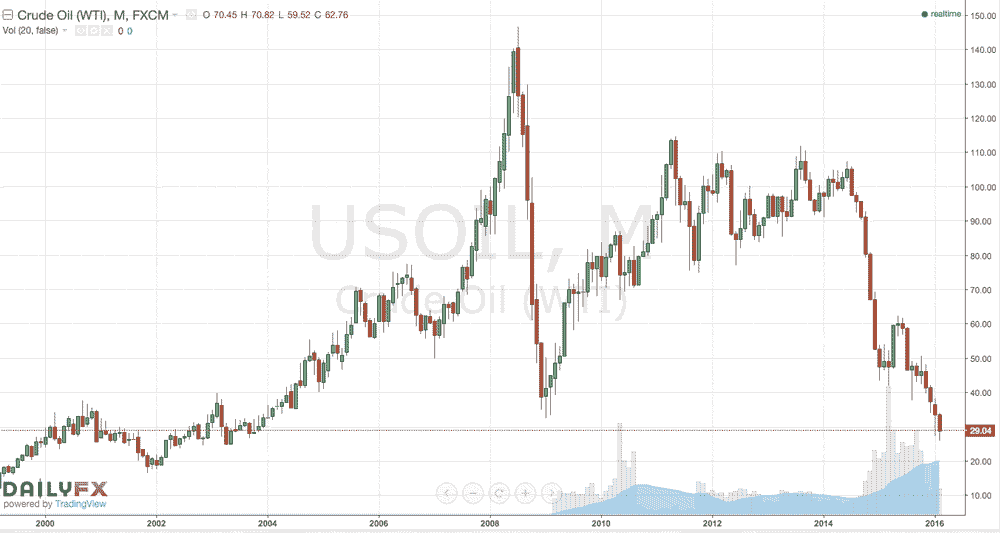
The price of operating an airline can fluctuate wildly. That's one of the reasons ticket prices change. A big reason for this is that air travel currently requires an enormous amount of fuel, roughly 4 litres per second of travel, and the cost of the oil used to make the fuel varies dramatically over time. If you're an airline company, you would be glad to have a way to control for these fluctuations a little. Here is a chart showing the the changes in the price of crude oil changed each month over the last 15 years.
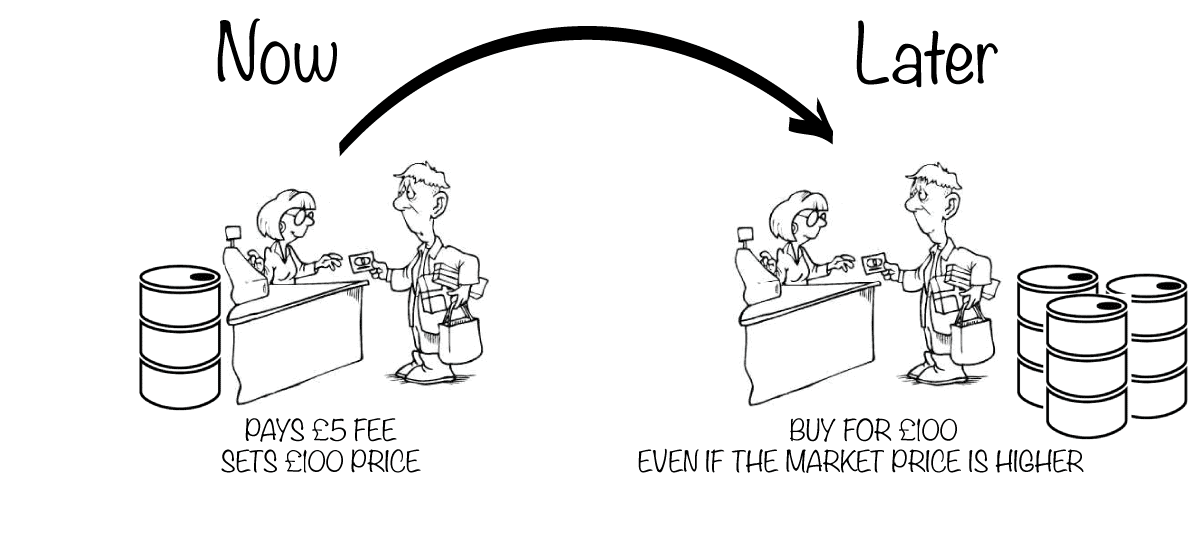
This problem solved through what is known as a futures contract. The oil company plans to produce 100 barrels of oil by the end of the year. So, it takes a gamble and offers the airline a contract to buy this oil at the current market price of £100 per barrel, but on 31 December. The airline is willing to pay a fee to enter into this contract, because it allows them to set their plane ticket prices for next month reliably even as oil prices fluctuate. And it provides the oil company with a guaranteed buyer. Everyone is happy.
Notice that determining the value of a futures contract requires knowing the price of another financial product, called the underlying financial product. In this case, the underlying is oil. So, a future is an example of a derivative.
Speculation: You aren't collecting oil
Most people involved in oil future contracts don't ever see any oil. They're doing it to make money. Futures contracts are sold in markets called futures exchanges, where you can purchase a futures contract because it includes a right to buy oil at a given price in the future. But it's not like you plan to ash in on that oil. The right to buy at a good price is worth money: if the price of oil on 31 December is £120 per barrel, and you have a contract to buy a barrel for £100, then you can sell your contract and make a profit of £20. In contrast, if the price of oil drops below £100, then you'll lose money.
From a speculator's perspective, future contracts are not as flexible as you might like. If the price of the underlying goes below your contract price, you might not wish to buy. To allow this possibility, another type of derivative was invented, called a call option. A call option is a security that provides its owner with the opportunity (but not an obligation) to buy something at a fixed price, called the strike price, at some time. In contrast, a put option provides its owner with an opportunity to sell something at a fixed price at some time.
For example, suppose that for £10 you purchase a call option to buy LinkedIn stock for £100, on 31 December. The price of LinkedIn stock may go up by that time, or it may go down:

If the price of the underlying LinkedIn shares go up to £150, then you are guaranteed to make money via the following clever deal: purchase your shares for £100 on 31 December, then sell them at market price for £150. This will leave you with a profit of,
\[ + £150 - £100 - £10 = £40 \]for each share that you purchase. In contrast, if the price of the stock drops below market price, you simply won't exercise your option, and will only lose the cost of your £10 fee.
We will return to derivatives, and especially options, in future lectures, as pricing these products provides one of the powerful applications of physics to finance. For now, let us just summarise the three derivative types we have seen so far.
| Derivative Type | What it gives you |
|---|---|
| Futures Contract | The right and obligation to buy (or sell) some agreed security at some future time. |
| Call option | The right (but no obligation) to buy at some agreed security for a fixed price at a future time. For a "European option" this is a single fixed date. For an "American option" the purchase can take place at any time up to a final fixed date. |
| Put option | The right (but no obligation) to sell some agreed security for a fixed price at a future time. The same concept of "European options" and "American options" applies here too. |
How do financial models represent the world?
A physicist's perspective on economics
In the beginning we pointed out that physics often proceeds by reducing a description to its basic states, together with the basic laws. For small systems, like a pair of planets, we can use what we know about the individual participants to make exact predictions about the future usin this technique.
However, for complex systems involving more than a few planets, keeping track of the individual participants is often hopeless. For example, notice how complex the behaviour of a simple double-pendulum can quickly become.