Imre Lakatos

In the last lecture we saw a radical new way to understand how scientific theories change. In fact, it was in many ways too radical. What was needed in the years following Kuhn's Structure was a more moderate and systematic account of scientific theory change.

That account was famously given by LSE philosopher of science Imre Lakatos. As often happens in philosophy, he began with a fresh and clear look at existing views, which led him to a new and more plausible alternative.

Imre Lakatos was originally not a philosopher, but a politician in communist Hungary in the 1940's. Like many of his fellow politicians, he was one day arrested and ended up in a forced labour camp for three years. On his release he began to study philosophy and mathematics. He fled Hungary during the revolution of 1956, and was able to study a PhD in Cambridge under the philosopher R. B. Braithwaite, although he was greatly influenced by a meeting with the mathematician George Pólya in 1958, whose work he had translated into English some years earlier.

Lakatos became a lecturer at LSE in 1960, joining Karl Popper in the Department of Philosophy, Logic & Scientific Method. Lakatos had an infectious excitement about him for the philosophy of science, and had an enormous influence on contemporary philosophy of science. You can listen to him speaking on the BBC below.

To understand the Lakatos world view, you have to first see how he understands his competitors. Those were, mainly: 1) the empiricists; 2) Kuhn; and 3) Popper. Let's discuss each of them in turn.

The Empiricist View: Inductivism

Paradigm Episode: Kepler and Tycho

A favourite episode for the empiricist view of theory change is that of Brahe and Kepler.

Tycho Brahe was a curious character. A dutch aristocrat born in 1546, he lost most of his nose in a swordfight over mathematics with a fellow student at age 20. Afterward he took to wearing a brass nose, carrying a little glue around with him in order to re-affix it to his face when needed.

At age 34 Tycho was invited to Prague to become the official imperial astronomer. There he collected meticulous astronomical data that was 30 times more accurate than the astronomical data that Copernicus had used. This helped him to develop a major geocentric alternative the Copernican (sun-centred) model of the Universe, now called the Tychonic system.

In 1600, a young German astronomer named Johannes Kepler came to Prague to visit and to assist Tycho in his measurements. Kepler naturally wanted to get his hands on the tables of data. Tycho was naturally protective of this valuable information and refused.

Kepler's luck turned when in 1601, Tycho waited too long to relieve himself at a dinner party and died some weeks later of a bladder infection. With Tycho gone, Kepler managed to steal the precious astronomical tables and use them for his own studies.

This new accurate data led Kepler in 1605 to formulate Kepler's laws, beginning with the claim that the planets follow elliptical orbits with sun in one focus.

What's particularly important about this episode for us is that Kepler began with the data and then correctly generalised it in order to discover his laws.

The inductivist perspective

It's helpful to separate the facts versus theory in describing these episodes.

According to the inductivist, drawing on the empiricist tradition, facts are elementary observations, such as the astronomical data collected by Tycho. And theories are inductive generalisations of those facts, and "correct" inductive generalisations at that. What is an inductive generalisation? Most crudely, it means that one takes some finite set of observations and infers a more general conclusion.

For example, one might infer from the fact that the sun has risen every morning so far that the sun always rises.

Lakatos likes to characterise each perspective on science by its code of honour. For the inductivist, that code consists in the rigour of accepting only facts and their inductive generalisations as science.

This does provide a coherent way to understand science. According to the inductivist, facts and their generalisations completely characterise science. The truth of a theory is assured by the correctness of the induction. And revolutions are nothing more than the unmasking of hidden errors in a theory, revealing that it was not a science after all but a pseudoscience.

Questions for the Inductivist

1. Self-Consistency One of the famous difficulties for the inductivist is the problem of self-consistency. Is the inductivist programme itself justified by basic facts, or by generalisation? If not, it would seem to be unscientific. This is similar to one of the concerns we noted for empiricism in the first week.

2. Choice of Facts? There's a lot of data out there in the world. It's absolutely teeming with it. But Kepler chose to examine one particular set of data, namely the astronomical tables of Tycho. He did not choose to study any other data, such as the tide tables or the behaviour of caterpillars.

Why does any scientist choose to study one set of data and not another? Inductivists lacks an internal explanation of this behaviour within their description of science. Their story is compatible with many possible external causes, such as the hypothesis that scientists choose what to study on the basis of social need (a view Lakatos refers to as "vulgar-Marxism"). But then we would lack a decent understanding of the apparent objectivity of science.

3. Why do errors occur so often? On the inductivist view, revolutions are due to human errors in reasoning. They occur because scientists sometimes act irrationally, believing things that are not warranted by the data or by inductive generalisation. But scientists are often wrong in this way. Why? Inductivism has no internal explanation of this either. It seems that it can only be explained in terms external to the inductivist account of science, such as through the psychology of the participants.

Kuhn's Approach: Conventionalism

Paradigm Episode: Copernican Revolution

As we saw in the last lecture, a classic episode for the Kuhnian picture of science is the Copernican revolution. It does not seem to have been brought on by a new accurate collection of data, by a crucial experiment, or by some failure of a previous theory.

Indeed, the Copernican and the Ptolemaic models appear to have been equally reasonable descriptions of the observed data when the Copernican model was proposed.

Kuhn's diagnosis is that it is an episode in which social influence played a crucial role. Lakatos calls this kind of response conventionalism.

Conventionalism: When members of a community agree to adopt scientific propositions and frameworks merely as a matter of convenience or convention.

Which aspects of science are taken to be conventions? There is a modest and a radical conventionalist response. The modest conventionalist takes facts and observations to be determined by the world rather than convention; theories, on the other hand, are frameworks (or pigeon-holes) for organising those observations, and they are a matter of convention. The radical conventionalist takes everything to be convention. This is perhaps closest to the view that Kuhn adopted, but is in danger of descending into radical skepticism about truth in science.

Truth on this view is tricky. It appears that theories can at best provide a rough indication of what truth is like. On the other hand, revolutions are very easy to describe: they occur when a community decides to abandon one convention system for another.

Questions for conventionalism

In terms of the "code of honour" that Lakatos likes to discuss, the conventionalist is unfortunately rather dishonourable. There is no rigorous principle determining what is a legitimate scientific framework and what is not. It is a view on which almost anything can in principle be counted as good science.

In the spirit of the previous questions, Lakatos also asks the following.

1. Choice of Facts? Like the inductivist, the modest conventionalist does not have an easy time describing why the scientist chooses one set of facts as opposed to any other. It is not part of the conventionalist description of science, and so is not internal to their story. And also like inductivism, it is perfectly compatible with a number of external descriptions, such as the suggestion that facts are chosen to study on the basis of social need.

2. Why do scientists lie? Scientists often report inferring a theory on the basis of empirical facts. This was the case with both Kepler and with Copernicus. But according to the conventionalist,, scientists are simply confused when they say this. What they are actually doing is just proposing a convention system in which to place the facts. Lakatos calls this false consciousness, and observes that it is a dislikeable feature of conventionalism.

Popper's Approach: Falsificationism

Paradigm example: Perihelion of Mercury

One of the major open problems in astronomy at the end of the 19th century was the understanding of the shifting perihelion of Mercury. By that I mean that the long axis of Mercury's elliptical orbit is not fixed, but slowly rotates round the sun at a rate of about 2 degrees per century.

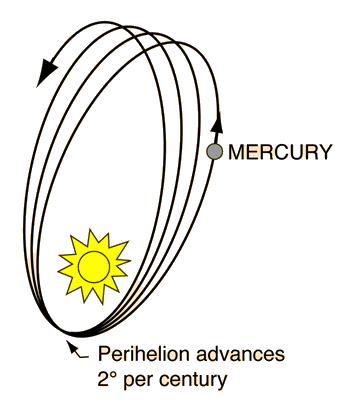
Scientists had tried to account for this for decades, by taking into account the effect of the orbits of Jupiter and Saturn, but the problem remained unsolved. Although scientists resisted it, they had managed to uncover evidence that falsified Newton's theory of gravity.

An alternative was found in Einstein's theory of general relativity, which provided a correct prediction of the anomalous orbit of mercury.

To check that Newton's theory had truly been overthrown, a further test was devised by the British physicist Arthur Eddington in May of 1919. Eddington took careful photographs during a solar eclipse of the locations of stars. Newtonian gravity predicted a straight path for light, whereas Einstein's theory predicted that the light would deviate slightly due to the curvature of spacetime.

Amazingly, Einstein found that Einstein's prediction was correct, and light really does bend slightly as it travels near the sun. And the experiment provided a further falsification of Newtonian gravity.

The falsificationist perspective

To the falsificationist, the only serious scientific statements are statements of falsification. Their conclusion about the episodes above is therefore about the failure of Newton rather than the success of Einstein.

The falsificationist perspective on fact and theory is almost complete reversal of the modest conventionalist perspective: nearly all factual or "basic" statements are adopted by convention; theoretical practice then consists in the construction of bold conjecture to attempt to falsify them. Correct scientific theory contains only descriptions of successful falsifications.

Like conventionalism, this view has it that theories can at best provide a rough indication of what truth is like, rather than a determination of the truth. It allows science to undergo revolutions, but on this view revolution means abandoning of one conjecture for another.

For the falsificationist, the "code of honour" was novelty.

Karl Popper emphasised this requirement repeatedly. Conjectures should not just aim to provide ad hoc solution to a problem. For example, some attempts to deal with Mercury's shifting perihelion involved changing Newton's law of gravity ever-so-slightly, by making the force of gravity proportional to 1/r^2.01 instead of 1/r².

To Popper and the falsificationists, this was very poor scientific practice. The paragon of good practice, on the other hand, was Einstein's bold conjecture that light would bend slightly as it passed near the sun. This was not an ad hoc attempt to describe existing observations, but a bold new conjecture about the nature of the world.

Questions for falsificationists

1. Why certain conjectures and not others? Like the other views, Popper has trouble explaining why scientists make certain conjectures and not others. Although Popper surely abhorred the idea, his perspective was even compatible with the vulgar-Marxist claim that scientific conjectures are determined by social need.

2. Scientists still lie? Popper also has to contend with the difficulty that scientists appear to report verifying theories. For example, the newspaper headline for Einstein's discovery says 'Einstein's theory triumphs' rather than 'Newton's theory fails'. According to Popper they are simply incorrect about this. So, Popper's description too has to deal with the problem of false-consciousness.

3. Definitive refutation? Finally, Popper had to contend with the difficulty that, just as it is so difficult to definitively verify a proposition, it seems also difficult to definitively refute one. If that's right, then falsificationism would seem to assert that science determines nothing at all, thus collapsing into the same skepticism as radical conventionalism.

Lakatos' Alternative: Scientific Research Programmes

Paradigm Episode: The anomalous orbit of Uranus

An earlier difficulty in 19th century gravitation was the problem of the orbit of Uranus. Extremely careful measurements of the location of Venus had been made by that time, and they did not agree with the prediction of Newton's theory given the locations of the known planets.

However, seemingly against the advice that Popper would have given, Newtonian gravity was not considered refuted. Instead, scientists scrambled to make sense of the anomalous orbit as best they could.

Convinced that there must be another planet causing the anomalous orbit, Urbain Le Verrier in France and John Couch Adams in England performed months of complex calculations in order to determine the exact orbit of a planet that would disturb Uranus in just the right way.

Le Verrier transmitted his results to the Berlin Observatory, where it was determined the same evening that a planet existed within 1 degree of the location that Le Verrier had predicted. Le Verrier had indeed discovered the planet Neptune.

The French physicist Francois Arago is said to have later referred to Le Verrier as "the man who discovered a planet with the point of his pen."

The Lakatos Perspective

The basic proposal of Lakatos is that the logic of such discoveries are best characterised by a scientific research programme, defined by the following properties.

• A hard core of claims that are taken for granted and never challenged. In the Neptune episode, an example of this would be Newton's law of gravity.
• A protective belt of further auxiliar assumptions that are more open to challenge than the hard core. Examples in the Neptune episode could include claims about the composition of the Earth's atmosphere, or the 19th century assumption that Uranus was the farthest planet from the sun.
• Positive heuristics that guide scientists as to which research path to pursue. For example, a positive heuristic might recommend that one critically explore the claim that Uranus is the farthest planet from the sun.
• Negative heuristics that guide scientists as to which research paths must not be pursued. For example, this would have advised 19th century astronomers not to challenge the hard core assumption of Newton's law of gravity.

On this picture protective belt serves like a moat. It gives scientists something to target and refine so as not to disturb the more central assumptions of the hard core. And like a moat, this protects the hard core from regular disturbance; this explains why science is so incredibly stable over the years.

This kind of idea nicely fits scientific practice. Lakatos himself wrote:

"if a planet does not move exactly as it should, the Newtonian scientist checks his conjectures concerning atmospheric refraction, concerning propagation of light in magnetic storms, and hundreds of other conjectures that are all part of the programme. He may even invent a hitherto unknown planet and calculate its position, mass and velocity in order to explain the anomaly."

It would be seemingly absurd to take Popper's advice in the case of Neptune. Instead, Lakatos counselled, one should view science as a research programme with a protective layer to prevent it from being falsified so easily.

One can moreover use these tools to describe the health of a scientific research programme in terms of its novel predictions.

In particular, Lakatos proposed that we examine the extent to which a theory is growing in the appropriate way. A theory will be called progressing as long as theoretical growth anticipates empirical growth (i.e. novel predictions are occurring) and some of those predictions are being corroborated by evidence. Otherwise, it is said to be stagnating or degenerate. In short, the number of novel predictions determine the health of a scientific research programme.

On the Lakatos picture of research programmes, theories grow in empirical predictions, and facts are collected to confirm or refute them. Success is measured by growth of theory over growth of facts. Truth is still a matter that is roughly indicated to us, rather than determined once and for all. And revolutions are replaced with "problemshifts": these occur when one research programme is replaced by another that is making more successful predictions. Thus in particular, falsification and anomaly are not required in order for theory change to occur on the Lakatos picture.

The code of honour for Lakatos is modesty. A research programme should not be viewed as so easily rejected as Popper would have it, nor should it be viewed as completely abandoned as Kuhn would say. Rather, research programmes tend to be shelved, and can certainly make a comeback. Thus, a scientific research programme must never presume that its competitors have disappeared. A shelved research programme may sometimes stage a comeback if the health of the alternative research programmes becomes sufficiently poor.

Some Lakatos distinctions

Sometimes just introducing such careful distinctions can be a helpful tool in the philosophy of science. Here are a few of the important ones that Lakatos makes. In his case, making these distinctions actually led him to a novel description of the logic of scientific discovery.

The picture Lakatos advocates of Scientific Research Programmes provide an internal explanation of why certain facts and conjectures are considered and not others: a positive heuristic guides us to them whenever we are involved in a research programme. One can also avoid the problem of false consciousness: scientists infer theories from facts when they say they do, and no alternative interpretation is needed.

But is it correct as a description of the logic of scientific discovery? Does it provide a reasonable description of the "paradigm examples" used to introduce the competing views? And what about Lakatos' description of progress: is mere growth of theory and novel predictions enough to be a true measure of success? I leave these questions to you.