1. Deduction vs. Induction

1.1. Deductive inferences in a scientific theory are inferences to the logical consequences of a theory. They are typically mathematical results that are guaranteed to be true whenever the assumptions of the theory are true.

Examples of Deductive Inferences:

1. Tommy is from Troy. (How dow we know this? We assume that Tommy is a Trojan and that Trojans are from Troy -- the conclusion follows as a matter of simple logic.)
2. In special relativity, the rate of clock in inertial motion with respect to some fixed frame of reference will be slower than the clocks in the fixed frame of reference. (Question: How do we know this?)
3. In special relativity, whether two spacelike-separated events are simultaneous depends on which inertial observer we ask. (Question: How do we know this?)

1.2. Inductive inferences in a scientific theory are that are not logical consequences of the theory, but which we nevertheless are warranted to infer.

Examples of Inductive Inferences:

1. The sun will rise tomorrow. (How do we know this? We know the sun rose yesterday, and the day before, and the day before. This makes it extremely likely -- though not a logical certainly -- that it will rise again tomorrow. In this sense, we are ``warranted'' in inferring the conclusion.)
2. There are no time travelers in our near future. (How might we argue this?)
3. There is 0 summed curvature above the surface of the earth.

2. Observationally indistinguishable spacetimes

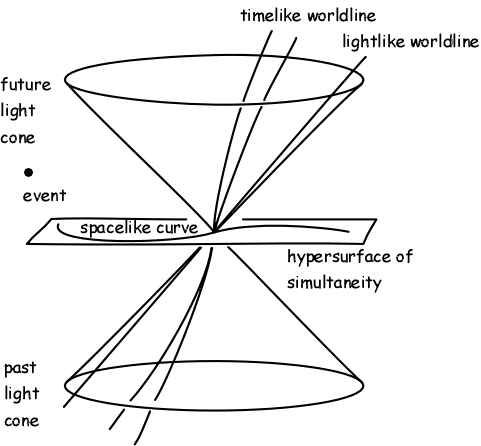
2.1. What can you know about the spacetime you're in? Answer: You can know at most everything in your past light cone.

More optimistically: You can aggregate information from a number of observers, including yourself, over the course of your lifetimes. Each ``observation'' will be a description of an observer's past light cone at a moment. Like this:

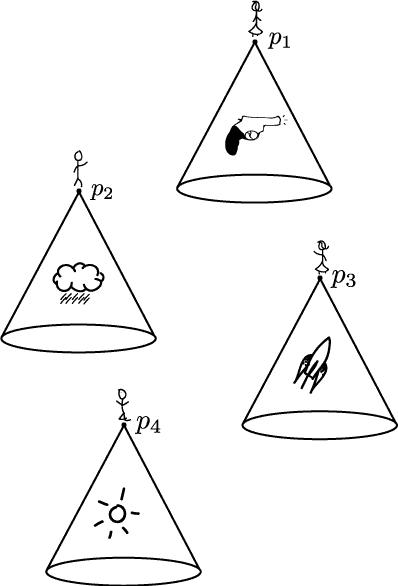
2.2. The problem is that no matter how many such observations you have, a recent discovery due to John Manchak shows that there are always at least two different spacetimes that are compatible with those observations. If a spacetime is such that any collection of its past light cones can be mapped onto a distinct spacetime in a way that preserves the distance relations on the light cones, then the two spacetimes are said to be Observationally indistinguishable.

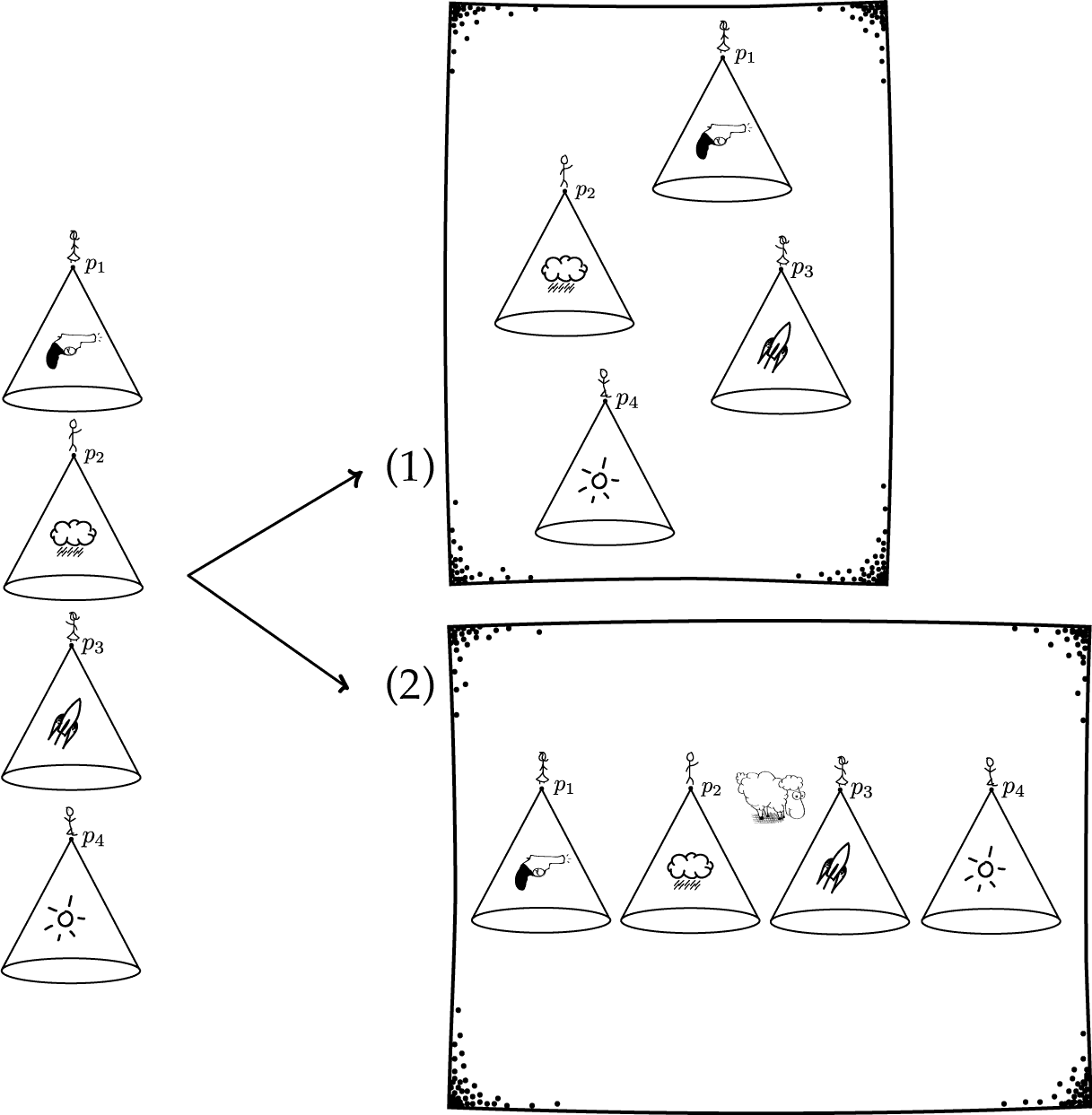
The most important result in this regard is Manchak's theorem: In spacetimes with no closed timelike curves, no number of observations is enough to know which spacetime we're in -- even assuming Einstein's equation and other local assumptions within general relativity!

3. Not known deductively, not known inductively

Because of the way this theorem works, it is not only the case that we cannot deductively determine which spacetime we're inhabit within the context of general relativity. But as Norton argues, our best attempts to inductively determine which spacetime we're in also seem to fail as a consequence of Manchak's theorem. Can you see why?

What you should know

• The difference between inductive and deductive inference and how to tell them apart.
• What observationally indistinguishable spacetimes are.
• What Manchak's theorem says.
• How it is that Manchak's theorem undermines any attempt to deductively infer which spacetime we're in.
• How it is that Manchak's theorem also seems to undermine attempts to inductively infer which spacetime we're in.